Whatdoesamagnifying glassdo to light

Normally magnifiers are expressed in terms of the magnifying power when \(L=\infty\) (case 3 above). For example, a magnifier with a power of 10 Diopter has a MP equal to \(2.5\) or \(2.5 \times\). In other words, the image is \(2.5\) times larger than it would be if the object would be at the near point of the unaided eye.

3. The object is at the focal point of the magnifier \(\left(s_{0}=f_{o}\right)\), so that the virtual image is at infinity \((L=\infty)\) and hence

How does magnifying glass worksphysics

In the Netherlands in the latter half of the 17th century, Antonie Philips van Leeuwenhoek created the single-lensed microscope (a microscope with one lens) and discovered microorganisms and sperm. At the same time in the United Kingdom, Robert Hooke created the compound microscope, with a combination of two lenses: an objective lens and an eyepiece, and used it to observe the structure of cork. Because the structure appeared to be a collection of small rooms like a beehive, he named these rooms cells. This led to the use of the word cell in biology. The currently used optical microscopes are generally compound microscopes that combine an objective lens and an eyepiece. With typical optical microscopes, the light source is located below the sample, and it is observed by using the objective lens to magnify the light that is transmitted through the sample. Therefore, it is not possible to observe objects that do not transmit light. To observe such samples, it is necessary to cut them into thin slices and secure these slices on glass slides or similar objects. Stereoscopic microscopes are used to observe objects that cannot be processed into thin slices. Stereoscopic microscopes are optical microscopes that project light down onto the sample. The reflected light is then magnified by the objective lens for observation. Stereoscopic microscopes have two eyepieces, allowing for 3D observation that is the same as viewing the sample with the naked eye. Stereoscopic microscopes are used for relatively low-magnification observation. Metallurgical microscopes are used to observe the light reflected from samples at high magnification.

10 uses ofmagnifying glassin laboratory

Optical microscope is the general term used for microscopes that use visual light and glass lenses to perform magnified observation of objects. Because they have historically been used to observe organisms such as microorganisms and the cells of plants and animals, they are also called biological microscopes.

\[\left.\operatorname{MP}\right|_{L=\infty}=d_{0} \mathfrak{D}, \nonumber \] for every distance \(l\) between the eye and the magnifying glass. The rays are parallel, so that the eye views the object in a relaxed way. This is the most common use of the magnifier.

A magnifying glass causes an image on the retina which is larger than without the magnifier. In principle, the image on the retina can be increased by simply bringing the object closer to the eye (reduce \(\left|s_{o}\right|\) at fixed \(s_{i}\) ). But \(\left|s_{o}\right|\) can not be smaller than the near point \(d_{o}\), which we take here to be \(25 \mathrm{~cm}\). It is desirable to use a lens that makes a magnified erect image at a distance to the eye greater than \(d_{o}\). This can be achieved by a positive lens with the object closer to the lens than the first focal point, thereby producing a magnified virtual image. An example is given in Figure \(\PageIndex{1}\).

10 uses ofmagnifying glass

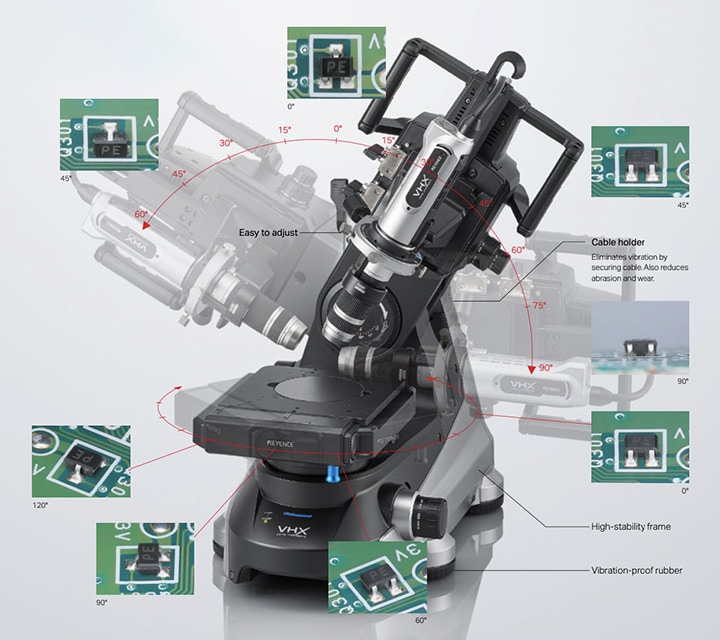
Optical microscopes are the most common type of microscope.By using an optical lens constructed of multiple objective lenses, they enable observations of the target at magnifications that are 100, 200, or 300 times higher than those performed with a microscope having a single lens.This section explains optical microscopes in detail.

It is said that the minimum distance between two points that can be distinguished with the naked eye is 0.1 mm, the thickness of a strand of hair. Optical microscopes can distinguish a minimum distance of 200 nm. Electron microscopes are generally used to observe objects smaller than 200 nm.

How doesamagnifying glasswork diagram

The magnifying power MP or angular magnification \(M_{a}\) is defined as the ratio of the size of the retinal image obtained with the instrument and the size of the retinal image as seen by the unaided eye at normal viewing distance \(d_{o}\). To estimate the size of the retinal image, we compare in both cases where the chief ray through the top of the object and the centre of the pupil of the eye hits the retina. Since the distance between the eye lens and the retina is fixed, the ratio of the image size on the retina for the eye with and without magnifying glass is: \[\mathrm{MP}=\frac{\alpha_{a}}{\alpha_{u}}, \nonumber \] where \(\alpha_{a}\) and \(\alpha_{u}\) are the angles between the optical axis and the chief rays for the aided and the unaided eye, respectively, as shown in Figure \(\PageIndex{2}\). Working with these angles instead of distances is in particular useful when the virtual image of the magnifying glass is at infinity. Using \(\alpha_{a} \approx y_{i} / L\) and \(\alpha_{u} \approx y_{0} / d_{0}\) with \(y_{i}\) and \(y_{0}\) positive and \(L\) the positive distance from the image to the eye (with as requirement : \(L \geq d_{o}\) ), we find \[\mathrm{MP}=\frac{y_{i} d_{0}}{y_{0} L} . \nonumber \] Since \(s_{i}<0\) and \(f_{o}<0\) we have, \[\frac{y_{i}}{y_{o}}=\frac{s_{i}}{s_{o}}=1+\frac{s_{i}}{f_{o}}, \nonumber \] where we used the lens equation for the magnifying glass. We have \(s_{i}=-\left|s_{i}\right|=-(L-\ell)\), where \(C\) is the distance between the magnifying glass and the eye. Hence, \(( \(\PageIndex{2}\) )\) becomes: \[\begin{aligned} \mathrm{MP} &=\frac{d_{0}}{L}\left[1+\frac{L-\iota}{\left|f_{o}\right|}\right] \\ &=\frac{d_{0}}{L}[1+\mathfrak{D}(L-\iota)], \end{aligned} \nonumber \] where \(\mathfrak{D}\) is the power of the magnifying glass.

3.6: Magnifying Glasses is shared under a not declared license and was authored, remixed, and/or curated by LibreTexts.

In practice \(d_{0} \mathfrak{D}=d_{o} /\left|f_{o}\right|\) is much larger than 1 , so that MP is similar in the three cases.

The LibreTexts libraries are Powered by NICE CXone Expert and are supported by the Department of Education Open Textbook Pilot Project, the UC Davis Office of the Provost, the UC Davis Library, the California State University Affordable Learning Solutions Program, and Merlot. We also acknowledge previous National Science Foundation support under grant numbers 1246120, 1525057, and 1413739. Legal. Accessibility Statement For more information contact us at info@libretexts.org.