In situations where the specimen is designed to be imaged without a coverslip, the working distance is measured at the actual surface of the specimen. Working distance typically decreases in a series of matched objectives as the magnification and numerical aperture increase. Objectives intended to view specimens with air as the imaging medium should have comparatively long working distances providing that numerical aperture requirements are satisfied. Alternatively, immersion objectives should have shallower working distances in order to keep the immersion liquid between the front lens and the specimen in place. Many objectives designed with similar working distances have a spring-loaded retraction stopper that allows the front lens assembly to be withdrawn by pushing it into the objective body and twisting to secure its place. Twisting the retraction stopper in the opposite direction releases the lens assembly for use. In some applications (see below), a long free working distance is indispensable, and special objectives are designed for such use despite how difficult it is to achieve large numerical apertures and the necessary degree of optical correction.

Laser Wavelength ChartExciton, c. 1980The origin of the laser was primed by the work of many, but Gordon Gould applied for a patent first in 1959, ...

Just as the brightness of illumination in a microscope is directed by the square of the working numerical aperture of the condenser, the brightness of an image produced by the objective is determined by the square of its numerical aperture. Additionally, objective magnification also plays a role in determining image brightness, which is inversely proportional to the square of the lateral magnification. The square of the numerical aperture/magnification ratio expresses the light-gathering power of the objective when used with transmitted illumination. High numerical aperture objectives collect more light and produce a brighter, more corrected image that is highly resolved because they also are often better corrected for aberration. In cases where the light level is a limiting factor (image brightness decreases rapidly as the magnification increases), choose an objective with the highest numerical aperture with the lowest magnification factor capable of producing sufficient resolution.

Polarization is a critical property of light for many optical systems and applications. This brief tutorial summarizes some of the most basic aspects of polarization, including how it is described, the impact of polarizing and birefringent elements on light, and how optical interfaces and filters can change the polarization of light.

The common design of a practical oil immersion objective includes a hemispherical front lens element, followed by a positive meniscus lens and a doublet lens group. Aplanatic refractions occur at the first two lens elements in a typical apochromatic oil immersion objective. Oil immersion objective lenses can also correct for chromatic defects that are introduced by the first two lens elements, while initiating a minimum amount of spherical aberration. Employing an oil immersion objective without oil between the cover slip and first lens element will result in defective images due to refraction that cannot be corrected by subsequent lens components within the objective.

Objectives typically have magnifying powers that range from 1:1 (1X) to 100:1 (100X), with the most common powers being 4X (or 5X), 10X, 20X, 40X (or 50X), and ...

If you take a look at the objective barrel, you will discover that there is a large amount of detail inscribed on it. Each objective is inscribed with the magnification; the tube length for which the objective was designed to give its finest images; and the thickness of coverslip protecting the specimen, which the designer assumed to have a constant value, correcting for spherical aberration. The objective will be engraved OIL or OEL or HI if the objective is designed to function with immersion oil. If not, the objective is meant to be used dry. Objectives are also always engraved with their numerical aperture value. If the objective does not indicate a higher correction, it is most likely an achromatic objective (more highly corrected objectives have inscriptions such as apochromat or apo, plan, FL, fluor, etc).

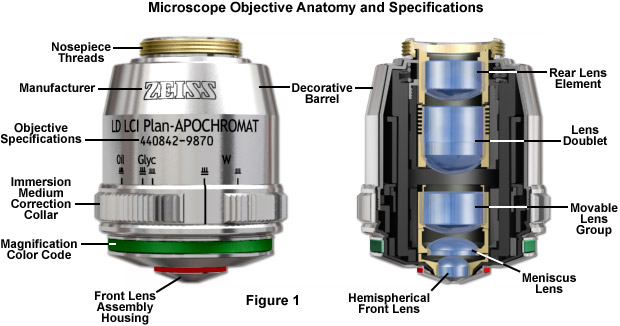
Major microscope manufacturers offer a wide range of objective designs that feature excellent optical characteristics under a wide spectrum of illumination conditions and provide various degrees of correction for the primary optical aberrations. The objective illustrated in Figure 1 is a 20x multi-immersion media plan-apochromat, which contains 9 optical elements that are cemented together into two groups of lens doublets, a movable lens triplet group, and two individual internal single-element lenses. The objective also has a hemispherical front lens and a meniscus second lens, which work synchronously to assist in capturing light rays at high numerical aperture with a minimum of spherical aberration. Many high magnification objectives are equipped with a spring-loaded retractable nosecone assembly that protects the front lens elements and the specimen from collision damage. Internal lens elements are carefully oriented and tightly packed into a tubular brass housing that is encapsulated by the decorative objective barrel. Specific objective parameters such as numerical aperture, magnification, optical tube length, degree of aberration correction, and other important characteristics are imprinted or engraved on the external portion of the barrel. The objective featured in Figure 1 is designed to operate utilizing water, glycerin, or a specialized hydrocarbon-based oil as the imaging medium.

When the objective is assembled, spherical aberration is corrected by selecting the best set of spacers to fit between the hemispherical and meniscus lens (the lower lens mounts). The objective is parfocalized by translating the entire lens cluster upward or downward within the sleeve with locking nuts so that focus will not be lost while objectives housed on a multiple nosepiece are interchanged. Adjustment for coma is accomplished with three centering screws that optimize the position of internal lens groups with respect to the optical axis of the objective.

LED UV is an emerging technology in print curing, promising long LED array lifetime and reduced energy consumption. Alpha-Cure is pleased to supply air and ...

When an optical filter is used at a non-normal angle of incidence, as is common with so-called “plate beamsplitters,” the filter can impact the polarization of the light. If the incident light is incoherent and unpolarized, and the optical system is “blind” to polarization, the standard intensity reflection and transmission functions R(l) and T(l) may be determined for the new angle of incidence, and they are sufficient to characterize the two emerging beams.

Other materials are nominally isotropic, but when they are bent or deformed in some way, they become anisotropic and therefore exhibit birefringence. This effect is widely used to study the mechanical properties of materials with optics.

Microscope manufacturers produce objectives with restricted tolerances to refractive index and dispersion. This means they require matching values in the liquid placed between the coverslip and objective front lens. It is advisable to employ only the oil intended by the objective manufacturer, and to not mix immersion oils between manufacturers. Additionally, objectives that use water and/or glycerin as an imaging medium are also available for applications with living cells in culture or sections of tissue immersed in physiological saline solution.

and where, as before, E = Ex< + Ey. The three special cases described in sections a, b, and c above thus correspond to: (a) Ax = Ay and  = 0 (linear polarization; equal amplitudes); (b) 

A majority of the microscope objectives being produced today offer extraordinarily low degrees of aberration and other imperfections, assuming the appropriate objective is selected and utilized properly. Even still, the microscopist must be conscious of the fact that objectives are not perfectly crafted from every standpoint, but are designed to meet a certain set of qualifications depending on intended use, constraints on physical dimensions, and price ranges. Consequently, objectives are made with degrees of correction that differ for chromatic and spherical aberration, field size and flatness, transmission wavelengths, freedom from fluorescence, birefringence, and additional factors contributing to background noise. Additionally, they are intended to be used under certain limited conditions, such as with particular tube lengths and tube lenses, type and thickness of immersion media and coverslips, wavelength ranges, field sizes, ocular types, and special condensers.

Fluorite objectives are fashioned from advanced glass formulations that contain materials such as fluorspar or newer synthetic substitutes that allow for greatly improved correction of optical aberration. Similar to the achromats, the fluorite objectives are also corrected chromatically for red and blue light, however, the fluorites are also spherically corrected for two or three colors instead of a single color, as are achromats. Compared to achromats, fluorite objectives are made with a higher numerical aperture, which results in brighter images. Fluorite objectives also have better resolving power than achromats and provide a higher degree of contrast, making them better suited for color photomicrography in white light.

Most polarizing beamsplitters are very efficient polarizers for the transmitted light (i.e., the ratio of desired to undesired polarization is very high); however, the reflected light generally contains some of both polarization components.How does a polarizer work? There are different ways of making a polarizer, and they are not described in detail here (see [1] for more examples). However, as an example consider one of the most popular absorbing polarizers: the well-known Polaroid “H-Sheet.” This polarizer, invented by E. H. Land in 1938, is a plastic, Poly-Vinyl Alcohol (PVA) sheet that has been heated and then stretched in one direction, forming long, nearly parallel hydrocarbon molecule chains. After dipping the sheet into an iodine-rich ink, long iodine chains form along the hydrocarbon molecules. Electrons freely move along the iodine chains, but do not easily move perpendicular to the chains. This ability for electrons to move freely in one direction but not the perpendicular direction is the key principle upon which most absorbing polarizers are based.

Electric polarization

Because the polarization response of a tilted multilayer thin-film coating can be very strong, optical filters can make excellent polarizers. For example, a basic edge filter at a high angle of incidence exhibits “edge splitting” – the edge wavelength for light at normal incidence shifts to a different wavelength for p-polarized light than it does for s-polarized light. As a result, there is a range of wavelengths for which p-polarized light is highly transmitted while s-polarized light ishighly reflected, as shown below.

A number 1½ coverslip is standard, with a thickness of 0.17 millimeters. Unfortunately, not all 1½ coverslips are manufactured to this standard (they range from 0.16 to 0.19 millimeters), and many specimens have media between them and the coverslip. By adjusting the mechanical tube length of the microscope, or by the utilization of specialized correction collars, compensation for coverslip thickness can be provided. Objective numerical aperture can be radically increased if the objective is used with an immersion medium such as oil, glycerin, or water. Typical immersion oils have a refractive index of 1.51 and a dispersion profile similar to that of glass cover slips. An immersion medium with a refractive index similar to that of the glass cover slip will practically eliminate image degradation due to thickness variations of the coverslip whereby rays of wide obliquity no longer undergo refraction and are more readily grasped by the objective. Light rays passing through the specimen encounter a homogeneous medium between the cover slip and immersion oil and are not refracted as they enter the lens, but only as they leave its upper surface. Therefore, if the specimen is placed at the aplanatic point of the first objective lens, imaging this portion of the lens system is totally free of spherical aberration.

Some polarizers eliminate the non-passed polarization component (Ey in the above example) by absorbing it, while others reflect this component. Absorbing polarizers are convenient when it is desirable to completely eliminate one polarization component from the system. A disadvantage  of absorbing polarizers is that they are not very durable and may be damaged by high intensity light (as found in many laser applications).When a reflective polarizer is operated in such a way that the blocked (i.e., reflected) polarization component is deflected into a convenient direction, such as 90° relative to the transmitted polarization component, then the polarizer acts like a polarizing beamsplitter, as shown below.

We can see that the tip of E traces out a circle as we follow the wave along the z axis at a fixed time. Similarly, if we sit at a fixed position, the tip of E appears to trace out a circle as time evolves. Hence this type of polarization is called circular polarization.

Jul 6, 2021 — Every light source requires an optic to work properly. According to Architecture Magazine, a compact fluorescent lamp can lose up to 70% of ...

Linear polarization example

The rear aperture or exit pupil of the objective restricts the light rays as they pass through an objective. The diameter of this aperture varies between 12 millimeters for low magnification objectives down to around 5 millimeters for the highest power apochromatic objectives. Close consideration of aperture size is absolutely imperative for epi-illumination applications that rely on the objective to act as both an imaging system and condenser, where the exit pupil also becomes an entrance pupil. The image of the light source must entirely fill the objective rear aperture to produce even illumination across the viewfield. If the light source image is smaller than the aperture, the viewfield will experience vignetting from uneven illumination. Conversely, if the light source image is larger than the rear aperture, all of the light will not enter the objective and the intensity of illumination is reduced.

It is also possible to take advantage of an appreciable difference in reflected or transmitted phase for p- and s-polarized light over a region of the spectrum where the reflected and transmitted intensities are essentially equal, thus forming a waveplate.

4.75" Round Dual LED Lighted Travel Makeup Mirror - Zadro: Portable, 10x Magnifying, Spot Clean, Battery Operated · Zadro · 3.8 out of 5 stars with 5 ratings. 5 ...

Representation of Electric Field E in Silicon Surface Barrier Detectors. Fig. 3. Silicon Detector Parameters Nomograph. [Similar to Nomogram reported by J. L..

If the difference between the two phase values is p/2, then the wave emerging from the material (say into air) will be circularly polarized. This occurs when

It is possible to correct for variations in coverslip thickness. Several high-performance apochromat dry objectives are fitted with correction collars that allow adjustment by a rotating collar, which causes two of the lens element groups in the objective to move closer together or farther apart (see Figure 4). Various specialized phase contrast objectives that are designed for tissue culture observation with an inverted microscope have an even broader compensation range of between 0 to 2 millimeters. In this way, specimens can be viewed through the bottom of most culture vessels, which in this size range, often have dramatic thickness fluctuations.

The most common objectives used on laboratory microscopes are the achromatic objectives. Such objectives are corrected for axial chromatic aberration in blue and red wavelengths, which are about 486 and 656 nanometers, respectively. Both are brought into a single common focal point. Achromatic objectives are also corrected for spherical aberration in the color green (546 nanometers; see Table 1). Achromatic objectives' limited correction can result in images with a magenta halo if focus is chosen in the green region of the spectrum. The lack of correction for flatness of field (or field curvature) presents a further problem. Plan achromats provide flat-field corrections for achromat objectives (Figure 2). An even higher level of correction and cost is found in objectives called fluorites or semi-apochromats (illustrated by center objective in Figure 2), named for the mineral fluorite, which was originally used in their construction.

Unpolarized light can be polarized using a “polarizer” or “polarizing beamsplitter,” and the state of already polarized light can be altered using a polarizer and/or optical components that are “birefringent.” In this section we explore some examples of these types of components.

Erin E. Wilson and Michael W. Davidson - National High Magnetic Field Laboratory, 1800 East Paul Dirac Dr., The Florida State University, Tallahassee, Florida, 32310.

Suppose the two components have equal amplitudes again, but now consider the case where these two components are not in phase, such that the angles of the sine functions are different. In particular, suppose there is a constant phase difference of p/2 between them, which corresponds to a distance of l/4 in the “fixed time” picture. The x component is

The third type of objective, the apochromatic objective, possesses the highest level of correction (Figure 2). Lower power apochromat objectives (5x, 10x, and 20x) have a longer working distance than higher power (40x and 100x) apochromat objectives. Apochromats almost eliminate chromatic aberration, are usually corrected chromatically for three colors (red, green, and blue), and are corrected spherically for either two or three wavelengths (see Table 1). Apochromatic objectives are the best choice for color photomicrography in white light. Because of their high level of correction, apochromat objectives usually have, for a given magnification, higher numerical apertures than do achromats or fluorites. Many of the newer high-performance fluorite and apochromat objectives are corrected for four (dark blue, blue, green, and red) or more colors chromatically and four colors spherically.

Polarization is a fundamental property of light. While many optical applications are based on systems that are “blind” to polarization, a very large number are not. Some applications rely directly on polarization as a key measurement variable, such as those based on how much an object depolarizes or rotates a polarized probe beam. For other applications, variations due to polarization are a source of noise, and thus throughout the system light must maintain a fixed state of polarization – or remain completely depolarized – to eliminate these variations. And for applications based on interference of non-parallel light beams, polarization greatly impacts contrast. As a result, for a large number of applications control of polarization is just as critical as control of ray propagation, diffraction, or the spectrum of the light. Yet despite its importance, polarization is often considered a more esoteric property of light that is not so well understood. In this article our aim is to answer some basic questions about the polarization of light, including: what polarization is and how it is described, how it is controlled by optical components, and when it matters in optical systems.

Elliptical polarization

The most important imaging component in the optical microscope is the objective, a complex multi-lens assembly that focuses light waves originating from the specimen and forms an intermediate image that is subsequently magnified by the eyepieces. Objectives are responsible for primary image formation and play a central role in establishing the quality of images that the microscope is capable of producing. Furthermore, the magnification of a particular specimen and the resolution under which fine specimen detail also heavily depends on microscope objectives. The most difficult component of an optical microscope to design and assemble, the objective is the first element that light encounters as it passes from the specimen to the image plane. Objectives received name from the fact that they are, by proximity, the closest component to the object, or specimen, being imaged.

Finally, the last but perhaps most important factor in determining the resolution of an objective is the angular aperture, which has a practical upper limit of about 72 degrees (with a sine value of 0.95). When combined with refractive index, the product:

Axiom Optics offers the latest technology in imaging and optical metrology. Your partner in SWIR cameras, laser beam profilers, wavefront sensors and more!

The amount of light output in each polarization state can be determined by simply breaking up the incident light into its two polarization components (s and p), and then calculating how much of each intensity is transmitted and reflected. For systems based on incoherent light, this level of detail is usually sufficient to keep track of the impacts of components like optical filters on polarization.For some optical systems – particularly those based on coherent light and that utilize or are sensitive to interference effects, for example – the complete state of polarization should be tracked at every point through the system. In that case, it is important to understand that optical filters based on multilayer thin-film coatings not only reflect and transmit different amounts of intensity for the s and p polarization states, but also impart different phases to the two different states. And both the amplitude and phase contributions can depend strongly on the wavelength of light. Thus, in general, an optical filter can act like the combination of a partial polarizer and a birefringent waveplate, for both reflected and transmitted light.To determine the effect of an optical filter on the light in such a system, the incident light should first be broken up into the two fundamental components associated with the plane of incidence of the filter (s and p components). Then, the amplitude and phase responses of the filter for the s and p components should be applied separately to each of the incident light components to determine the amplitudes and phases of the reflected and transmitted light components. Finally, the reflected s and p components can be recombined to determine the total reflected light and its state of polarization, and likewise for the transmitted light. These steps are illustrated in the diagram below.

where Resolution is the minimum separation distance between two point objects that are clearly resolved, λ is the illumination wavelength, n is the imaging medium refractive index, and θ is equal to one-half of the objective angular aperture. With this in mind, it is apparent that resolution is directly proportional to the illumination wavelength. The human eye responds to the wavelength region between 400 and 700 nanometers, which represents the visible light spectrum that is utilized for a majority of microscope observations. Resolution is also dependent upon the refractive index of the imaging medium and the objective angular aperture. Objectives are intended to image specimens either through air or a medium of higher refractive index between the front lens and the specimen. The field of view is often highly restricted, and the front lens element of the objective is placed close to the specimen with which it must lie in optical contact. A gain in resolution by a factor of about 1.5 is attained when immersion oil is substituted for air as the imaging medium.

For many years, field curvature went uncorrected as the most severe optical aberration that occurred in fluorite (semi-apochromat) and apochromat objectives, tolerated as an unavoidable artifact. The introduction of flat-field (plan) correction to objectives perfected their use for photomicrography and video microscopy, and today these corrections are standard in both general use and high-performance objectives. Figure 3 illustrates how correction for field curvature (for a simple achromat) adds a considerable number of lens elements to the objective. The significant increase in lens elements for plan correction also occurs with fluorite and apochromat objectives, frequently resulting in an extremely tight fit of lens elements (see Figure 1) within the internal objective sleeve.

Some materials have a different index of refraction for light polarized along different directions. This phenomenon is called birefringence. For example, suppose light polarized along the x direction sees an index of nx, while light polarized along the y direction sees an index ny. Now suppose linearly polarized light passes through a piece of such a material of length L, where the linear polarization axis is oriented at 45° with respect to the x and y axes. The fixed time picture thus looks like:

A polarizer transmits only a single orientation of linear polarization, and blocks the rest of the light. For example, a polarizer oriented along x passes x and blocks Ey.

All three types of objectives suffer from pronounced field curvature, thus they project curved images rather than flat ones. Such artifact increases in severity with higher magnification. To overcome this inherent condition, optical designers have produced flat-field corrected objectives, which yield images that are in common focus throughout the viewfield. Objectives that have flat-field correction and low distortion are called plan achromats, plan fluorites, or plan apochromats, depending upon their degree of residual aberration. This correction, although expensive, is extremely valuable in digital imaging and conventional photomicrography.

All of the states of polarization described above are actually special cases of the most general state of polarization, called elliptical polarization, in which the tip of the electric field vector E traces out an ellipse in the x-y plane. The two components might have unequal amplitudes Ax  Ay , and also might contain a different relative phase, often denoted  That is, we may write generally

Circularlypolarized light

Clamps & Mounting Brackets. A variety of camera clamps, mounting brackets, arms and spigots. Great for attaching cameras to a variety of surfaces including ...

There are three vital design characteristics of the objective that set the ultimate resolution limit of the microscope: The wavelength of light used to illuminate the specimen, the angular aperture of the light cone captured by the objective, and the refractive index in the object space between the objective front lens and the specimen. Resolution for a diffraction-limited optical microscope can be described as the minimum visible distance between two closely spaced specimen points:

In many biological and petrographic applications, when mounting the specimen, a glass coverslip is used to both protect the integrity of the specimen and to provide a clear window for observation. The coverslip acts to converge the light cones originating from each point in the specimen. But it also introduces chromatic and spherical aberration that must be corrected by the objective. The refractive index, dispersion, and thickness of the coverslip determine the degree to which light rays are converged. An additional concern is the aqueous solvent or excess mounting medium that lies between the specimen and coverslip in wet or thickly mounted preparations, which add to the variations in refractive index and thickness of the cover slip.

We can see that in general the light emerges in a different state of elliptic polarization. In fact, for the example illustrated above, the particular choice of L for a given difference between nx and ny causes the linearly polarized light at the input end to be converted to circularly polarized light at the other end of the birefringent material. How did this happen? Let’s look at the math. Consider the phases accumulated by the two component waves as they travel through the birefringent material. The waves can be described by

What if the two components Ex and Ey have unequal amplitude factors? We can see that the light wave is still linearly polarized.

Older objectives typically have lower numerical apertures, and are subject to chromatic difference of magnification, an aberration that requires correction by the use of specially designed compensating oculars or eyepieces. This type of correction was prevalent during the popularity of fixed tube length microscopes, but is not necessary with modern infinity-corrected objectives and microscopes. Recently, correction for chromatic difference of magnification is either built into the modern microscope objectives themselves (Olympus and Nikon), or corrected in the tube lens (Leica and Zeiss). The intermediate image in an infinity-corrected system appears behind the tube lens in the optical pathway at the reference focal length. The tube lens focal length varies between 160 and 250 millimeters, depending upon design constraints imposed by the manufacturer. By dividing the reference focal length by the focal length of the objective lens, the magnification of an infinity-corrected objective can be calculated.

Circular polarization

The polarization of light reflected and transmitted at an interface between two media or at a thin-film multilayer coating can be altered dramatically. These two cases are considered below.

is known as the numerical aperture (NA), and provides an important indicator of the resolution for any particular objective. Other than magnification, numerical aperture is generally the most important design criteria when considering which microscope objective to choose. Values range from 0.025 for very low magnification objectives (1x to 4x) to as much as 1.6 for high-performance objectives that employ specialized immersion oils. As numerical aperture values increase for a series of objectives of the same magnification, a greater light-gathering ability and increase in resolution occurs. Under the best circumstances, detail that is just resolved should be enlarged sufficiently to be viewed with comfort, but not to the point that empty magnification obstructs observation of fine specimen detail. The microscopist should carefully choose the numerical aperture of an objective to match the magnification produced in the final image. Magnifications higher than this value will yield no additional useful information (or finer resolution of image detail), and will lead to image degradation. Exceeding the limit of useful magnification causes the image to suffer from empty magnification, where increasing magnification will simply cause the image to become more magnified with no corresponding increase in resolution.

That is, E appears to oscillate along a line oriented at 45° with respect to the x axis. Hence this situation is called linear polarization.Notice that equivalently we could view the wave at a particular location (“fixed position”) and watch its amplitude evolve with time. Suppose we sit at the position z = 0. Then we see that

The amplitude E, or the potential for a charged particle to feel a force, is vibrating along both the x and y directions. An actual charged particle would feel both of these fields simultaneously, or it would feel

In other words, if we look down the propagation axis in the positive x direction, the vector E at various locations (and at t = 0) now looks like:

When the electric field of a light wave encounters the sheet, the component parallel to the chains causes electrons to oscillate along the direction of that component (Ey in the above example), thus absorbing energy and inhibiting the component from passing through the sheet. Because electrons can not respond to the other component (Ex), it is readily transmitted.

For several years, most manufacturers conformed to an international standard of parfocal distance when designing objective lenses for biological applications. As a result, a majority of objectives had a parfocal distance of 45.0 millimeters and were considered interchangeable. As it became commonplace to produce infinity-corrected tube lengths, a new set of design criteria was created to correct for aberrations in the objective and tube lenses. Alongside a demand for greater flexibility to accommodate the requirement of expanding working distances with higher numerical apertures and field sizes, interchangeability between objective lenses from different manufacturers is now more limited.

However, if the optical system is in any way sensitive to polarization, even when the incident light is unpolarized, it is important to recognize that the beamsplitter can transmit and reflect different amounts of the “s” and “p” polarization states, as shown below.

A dramatic improvement in contrast and transmission of visible wavelengths is the result of most microscope manufacturers currently producing their own proprietary formulations, along with a simultaneous destructive interference in harmonically-related frequencies lying outside the transmission band. The microscopist should be aware of the fact that these specialized coatings can be easily damaged by mis-handling. A good rule to employ in order to distinguish between coatings is that multilayer antireflection coatings have a slightly greenish tint, as opposed to the purplish tint of single-layer coatings. Also, the surface layer of antireflection coatings used on internal lenses is often much softer than corresponding coatings. Special care should be taken when cleaning optical surfaces that have been coated with thin films, especially if the microscope has been disassembled and the internal lens elements are subject to inspection.

Notice from the graph above on the right that for the case of reflection from a higher-index region to a lower-index region (in this case glass-to-air, or ni = 1.5 and nt = 1.0), the reflectivity becomes 100% for all angles greater than the “critical angle” θc = arcsin(nt/ni) and for both polarizations. This phenomenon is known as “Total Internal Reflection” (TIR).For angles of incidence below the critical angle only the amplitudes of the different polarization components are affected by reflection or transmission at an interface. Except for discrete changes of p (or 180°), the phase of the light is unchanged. Thus, the state of polarization can change in only limited ways. For example, linearly polarized light remains linearly polarized, although its orientation (angle ) may rotate. However, for angles greater than θc, different polarizations experience different phase changes, and thus TIR can affect the state of polarization of a light wave in the same way birefringence does. Thus linearly polarized light may become elliptical, or vice versa, in addition to changes in the orientation.

The angle of the reflected ray,θr, is always equal to the angle of the incident ray, θi, this result is called the “law of reflection.” The angle of the transmitted (or refracted) ray, θT, is related to the angle of incidence by the well-known “Snell’s Law” relationship: ni sin θinbsp;= nt sin θT. It turns out that s-polarized light is always more highly reflected than p-polarized light. In fact, at a special angle called “Brewster’s Angle,” denoted θB, the p-polarized component sees no reflection, or is completely transmitted. Brewster’s angle is given by θB = arctan(nt/ni). The power or intensity reflection coefficients for a light wave (i.e., the squares of the amplitude reflection coefficients) for air-to-glass (left) and glass-to-air (right) look like:

If Ax  Ay , the total wave E is linearly polarized, but it is no longer oriented at 45° with respect to the x axis. In fact we can see that it is oriented at an angle  where

polarization中文

When light is incident on an interface between two different media with different indexes of refraction, some of the light is reflected and some is transmitted. When the angle of incidence is not normal, different polarizations are reflected (and transmitted) by different amounts. This dependence was first properly described by Fresnel, and hence it is often called “Fresnel Reflection.” It is simplest to describe the polarization of the incident, reflected, and transmitted (refracted) light in terms of a vector component perpendicular to the plane of incidence, called the “s” component, and a component parallel to the plane of incidence, called the “p” component. The “plane of incidence” is the plane which contains the incident ray and the transmitted and reflected rays (i.e., all of these rays lie on one plane). In the example in the diagram below, the plane of incidence is the plane containing the x and z axes. That is, Es || y, while Ep lies in the x-z plane.

To understand the polarization of light, we must first recognize that light can be described as a classical wave. The most basic parameters that describe any wave are the amplitude and the wavelength. For example, the amplitude of a wave represents the longitudinal displacement of air molecules for a sound wave traveling through the air, or the transverse displacement of a string or water molecules for a wave on a guitar string or on the surface of a pond, respectively. We will refer to the amplitude of a light wave with the letter “E.” The amplitude of a light wave represents the potential for a charged particle (such as an electron) to feel a force – formally it may represent the “electric field” of an electromagnetic wave. Because this potential vibrates along the directions transverse to the direction the wave is traveling, light is a “transverse wave,” just like the waves on a string or water surface.Because light is a transverse wave, and because there are two transverse dimensions, there are fundamentally two distinct directions in which the light wave may oscillate. Let’s call these the x and y directions for a light wave traveling along the z direction. We’ll call the two distinct waves Ex and Ey, where we denote these by vectors to remind us that they point in (or oscillate along) a certain direction (the x and y directions, respectively).The amplitude of the light wave describes how the wave propagates in position and time. Mathematically, we can write it as a “sine wave” where the angle of the sine function is a linear combination of both position and time terms:

Polarization

The imaging medium between the objective front lens and the specimen cover slip is another important element in respect to correction for spherical aberration and coma in the design of lens elements for objectives. Lower power objectives are designed to be used with only air as the imaging medium between the objective front lens and the coverslip. The maximum theoretical numerical aperture obtainable with air is 1.0, however in practice it is virtually impossible to produce a dry objective with a numerical aperture above 0.95. The effect of coverslip thickness variation is negligible for dry objectives having numerical apertures less than 0.4, but such deviation becomes significant at numerical apertures exceeding 0.65, where fluctuations as small as 0.01 millimeter can introduce spherical aberration.

Multilayer thin-film coatings have a large number of interfaces, since they are generally comprised of alternating layers of a high- and low-index layer materials. The fraction of incident light intensity Iin that is reflected (IR) and transmitted (IT) through a thin-film coating can be calculated from the indexes of refraction and the precise thicknesses of each layer. These intensity reflection and transmission functions R(l) and T(l), respectively, generally depend strongly on the wavelength of the light, because the total amount of light reflected from and transmitted through the coating comes from the interference of many individual waves that arise from the partial reflection and transmission at each interface. That is why optical filters based on thin-film coatings are called “interference filters.”

Because of this relationship, a material with birefringence Dn of the appropriate thickness L to convert linear polarization to circular polarization is called a quarter-wave plate.What causes materials to be birefringent? Some materials, especially crystals, are naturally anisotropic at microscopic (sub-wavelength) size scales. For example, Calcite (CaCO3) is shown in the drawing below. The structure, and hence the response to polarized light, along the c direction is markedly different than that along the a and b directions, thus leading to a different index of refraction for light polarized along this direction.

Polarization oflight

In the past 100 years, construction techniques and materials used to manufacture objectives have greatly improved. Composed up of numerous internal glass lens elements, modern objectives have reached a high state of quality and performance considering the extent of correction for aberrations and flatness of field. Objectives are currently designed with the assistance of Computer-Aided-Design (CAD) systems, which use advanced rare-element glass formulations of uniform composition and quality characterized by highly specific refractive indices. These advanced techniques have allowed manufacturers to produce objectives that are very low in dispersion and corrected for most of the common optical artifacts such as coma, astigmatism, geometrical distortion, field curvature, spherical and chromatic aberration. Not only are microscope objectives now corrected for more aberrations over wider fields, but image flare has been dramatically reduced thanks to modern coating technologies, with a substantial increase in light transmission, yielding images that are remarkably bright, sharp, and crisp.

where A is called the “amplitude factor,” the variable l (“lambda”) is the “wavelength” (units of nm), and the variable v (“nu”) is the “frequency” (units of Hz, or sec–1). If a snapshot of the wave could be taken at a fixed time, l would be the distance from one wave peak to the next. If one sits at a fixed point in space and counts the wave peaks as they pass by, v gives the frequency of these counts, or 1/v gives the time between peaks. The sign between the position and time terms determines the direction the wave travels: when the two terms have the opposite sign (i.e., the “–” sign is chosen), the wave travels in the positive z direction. For convenience we often use two new variables called the “wavenumber” k = 2p/l and the “angular frequency” 2pv (“omega”), which absorb the factor of 2p, so that the wave amplitude can now be written more compactly as

Specifically, RGB images are obtained on the R/G/B channels, multispectral images are obtained at a small number of discontinuous wavelengths, and hyperspectral ...

Using this description of a single transverse orientation of a light wave, we can now consider multiple orientations to describe different states of polarization.

Meiji EM-30 Dual Magnification Stereo Microscope Series.

The distance from the lens center to a point where parallel rays are focused on the optical axis is defined as the focal length of a lens system. An imaginary plane perpendicular to the principal focal point is called the focal plane of the lens system. There are two principal focal points, one in front and one at the rear, for light entering each side of every lens. Conventionally, the objective focal plane found nearer to the front lens element is known as the front focal plane and the focal plane located behind the objective is known as the rear focal plane. The specific position of the rear focal plane varies with construction of the objective, but is usually situated somewhere inside the objective barrel for high magnification objectives. Lower magnification objectives often have a rear focal plane that is located on the exterior, in the thread area or within the microscope nosepiece.

One of the most significant improvements in objective design during recent years is the enhancement of antireflection coating technology, which aides in reducing unnecessary reflections that occur as light passes through the lens system. Each uncoated air-glass interface is capable of reflecting between four and five percent of an incident light beam normal to the surface, resulting in a transmission value of 95-96 percent at normal incidence. If a quarter-wavelength thick antireflection coating with the appropriate refractive index is applied, it can increase this value by three to four percent. Multilayer coatings, which produce transmission values exceeding 99.9 percent in the visible spectral range, have replaced the single-layer lens coatings once used to reduce glare and improve transmission.