does not exhibit any lower limit on the wavelength of propagating light at a given frequency. Thus, similar to the 2D optics of surface plasmon polaritons, there is no usual diffraction limit in this metamaterial medium. Abbe’s resolution limit simply does not exist. Optical energy propagates through such metamaterial in the form of radial rays, as shown in Fig. 1. If point sources are located near the inner rim of the concentric metamaterial structure, the lateral separation of the rays radiated from these sources would increase upon propagation towards the outer rim. Resolution of an “immersion” microscope based on such a metamaterial structure is defined by the ratio of inner to outer radii. Resolution appears limited only by losses, which can be compensated by optical gain.

Diffraction limitexplained

Secure .gov websites use HTTPS A lock ( Lock Locked padlock icon ) or https:// means you've safely connected to the .gov website. Share sensitive information only on official, secure websites.

Over the past few years two major new thrusts have developed in optical microscopy that are quickly demolishing the resolution barrier due to the diffraction limit. The first one is making use of nonlinear optics. A comprehensive review of this major research thrust has been published very recently by Hell (2007). Broadly speaking, these techniques rely on photoswitching and∕or saturation of fluorescence from individual molecules. They demonstrate far-field resolution of 20 to 30 nm, which is limited by light collection. The latest exciting example of these techniques has been demonstrated by the Zhuang’s group from the Harvard University (Huang et al., 2008). They have used the so-called stochastic optical reconstruction microscopy (STORM) technique supplemented by additional optical astigmatism in the optical path in order to determine both axial and lateral positions of individual fluorophores with nanometer accuracy. The major achievement of this work is that ∼20 nm lateral resolution is supplemented by ∼50 nm resolution in the axial direction, which allowed them to reconstruct complete 3D images, without scanning the sample. This development allowed the group to resolve the 3D morphology of nanoscopic cellular structures, such as clathrin-coated pits in a cell. These experiments have demonstrated the ability of 3D STORM technique to resolve nanoscopic features of cellular structures with molecular specificity under ambient conditions.

Diffraction limit of lightmicroscopy

Most people use put a transparent plastic ruler under microscope to perform this task. Actually, the microscope itself provides pretty accurate measurements ranging from 0.2 - 10 mm. Based on optical physics, the diameter of the field of view can be reliably derived by a simple formula:

Official websites use .gov A .gov website belongs to an official government organization in the United States.

Diffraction limit of lightcalculator

A parallel revolutionary development in usual linear optical microscopy was inspired by a seminal paper by Pendry (2000) and the following extraordinary progress in the optics of metamaterials. According to the Pendry’s idea of a flat “perfect lens” made from an artificial negative refractive index (meta)material, a high-resolution optical image could be obtained by amplified evanescent waves (surface plasmon polaritons) that live at the interface between the positive and negative index media. However, according to the original proposal, such an image would be observable only in the near-field of a perfect lens, and would require an auxiliary near-field microscope. Indeed, imaging of this kind had been reported in 2005 in two independent experiments performed by Zhang’s group from Berkeley (Fang et al., 2005) and Blaikie’s group from the Canterbury University in New Zealand (Melville and Blaikie, 2005). Nevertheless, until recently this technique was limited by the fact that magnification of the planar superlens is equal to 1.

Optical microscopy is one of the oldest research tools. It dates back to 1609 when Galileo Galilei developed an occhiolino, or compound microscope with a convex and a concave lens. Although various electron and scanning probe microscopes have long surpassed it in resolving power, optical microscopy remains invaluable in many fields of science. The reason for the limited resolution of an optical microscope is diffraction and, ultimately, the uncertainty principle: a wave cannot be localized much tighter than half of its vacuum wavelength, λ∕2. Immersion microscopes introduced by Abbe in the 19th century have slightly improved resolution on the order of λ∕2n because of the shorter wavelength of light, λ∕n, in a medium with refractive index, n. However, immersion microscopes are limited by the small range of refractive indices, n, of available transparent materials. For a while it was believed that the only way to achieve nanometer-scale spatial resolution in an optical microscope is to detect evanescent optical waves in very close proximity to a studied sample using a scanning near-field optical microscope (Phol and Courjon, 1993). Although many fascinating results are being obtained with near-field optics, such microscopes are not as versatile and convenient to use as regular far-field optical microscopes. For example, an image of a near-field optical microscope is obtained by point-by-point scanning, which is an indirect and a rather slow process.

Diffraction limit of lightformula

For example: Diameter of  the field of view (mm) = 20 / 40 = 0.50, where 20 is the field number of eyepiece, and 40 = objective mag.

Diffraction limitresolution

where ϵd is the dielectic constant of the medium bounding metal surface, kxy=kp is the wave vector component in the plane of propagation, and kz is the wave vector component perpendicular to the plane. This form of the dispersion relation originates from the exponential decay of the surface wave field away from the propagation plane. Negative refractive index behavior of surface plasmons was also shown to play a very important role in these early experiments (Smolyaninov et al., 2005b).

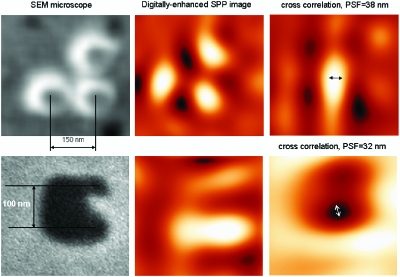
Following these theoretical ideas, magnifying superlenses (or hyperlenses) were independently realized in two experiments (Smolyaninov et al., 2007; Liu et al., 2007). Far-field optical resolution of at least 70 nm has been demonstrated using a magnifying superlens based on a 2D plasmonic metamaterial design (Smolyaninov et al., 2007). Using the experimentally measured point spread function of the microscope, resolution of plasmon microscopy may be further improved to ∼30 nm scale (Fig. 2) by implementing digital resolution enhancement techniques (Smolyaninov et al., 2006). Thus, it appears that both major thrusts in far-field optical microscopy: the nonlinear super-resolution techniques (Hell, 2007) and the linear techniques based on plasmonic and optical metamaterials, are quickly moving the resolution scale of far-field optical microscopy towards the 10 nm level. Widespread availability of these techniques to the research community should bring about numerous revolutionary advances in biomedical imaging.

Most modern microscopes have eyepieces with the number of field of view at 20 or 22. The diameters of the field of view are listed:

Abbediffraction limitderivation

Over the past century the resolution of far-field optical microscopes, which rely on propagating optical modes, was widely believed to be limited because of diffraction to a value on the order of a half-wavelength λ∕2 of the light used. Although immersion microscopes had slightly improved resolution on the order of λ∕2n, the increased resolution was limited by the small range of refractive indices, n, of available transparent materials. We are experiencing quick demolition of the diffraction limit in optical microscopy. Over the past few years numerous nonlinear optical microscopy techniques based on photoswitching and saturation of fluorescence demonstrated far-field resolution of 20 to 30 nm. The latest exciting example of these techniques has been demonstrated by Huang et al. [Science 319, 810–813 (2008)]. Moreover, recent progress in metamaterials indicates that artificial optical media can be created, which do not exhibit the diffraction limit. Resolution of linear “immersion” microscopes based on such metamaterials appears limited only by losses, which can be compensated by gain media. Thus, optical microscopy is quickly moving towards the 10 nm resolution scale, which should bring about numerous revolutionary advances in biomedical imaging.

Diffraction limitcalculator

Diameter of the field of view (mm) = F / M, where F is the number of field of view (FOV) of the eyepiece, and M is the magnification (mag.) of the objective.

Near the edge of the superlens the separation of three rays (marked by arrows) is large enough to be resolved using a conventional optical microscope. (b) Theoretical simulation of ray propagation in the magnifying superlens microscope.

The field area (A) is calculated by . If the cell is used as unit (instead of mm), the total number of cells in the field of view can be derived from the number of cells on the diameter line with the formula. This is an excellent approximation for most of our histological samples.  For example, if 16 positive cells and 28 total cells are on the line across diameter, respectively. Then Positive cells (Pi x 82 =201) divided by total cells number (Pi x 142 = 616) in entire field would be % of positive cells (201/ 616= 32.6%) (Figure).

On the theoretical side, various new geometries exhibiting image magnification beyond the usual diffraction limit were proposed (Ramakrishna and Pendry, 2004; Jakob et al., 2006; Salandrino and Engheta, 2006), which make use of newly developed optical metamaterials. For example, in the “optical hyperlens” design developed by Narimanov’s group (Jakob et al., 2006), an optical metamaterial made of a concentric arrangement of metal and dielectric cylinders may be characterized by a strongly anisotropic dielectric permittivity tensor in which the tangential ϵθ and the radial ϵr components have opposite signs. The resulting hyperbolic dispersion relation,

An important early step to overcome this limitation was made in surface plasmon-assisted microscopy experiments (Smolyaninov et al., 2005a), in which two-dimensional (2D) image magnification has been achieved. The increased spatial resolution of microscopy experiments performed with surface plasmon polaritons (Zayats and Smolyaninov, 2003) is based on the “hyperbolic” dispersion law of such waves, which may be written in the form