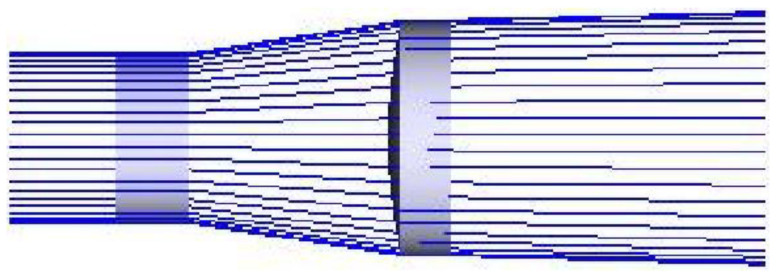
Two cylindrical lenses were used to shape the X and Y directions, and the lenses did not interfere with each other. Thus, after the two cylindrical lenses were individually designed, they could simply be stacked. The refraction–surface radius, air thickness, nonlinear coefficient of the aspheric surface, and 4th–10th order coefficients were set as the optimization variables for the system. The distance between the shaping lenses for the X- and Y-directions was set to 142 mm. The optimized system structure is shown in Table 1.

We adopted the optimized design method using the combined optical components, as described in Section 3.2, and used approximately 20% of the light-intensity difference between the center of the spot and the edge as the adjustment range. We calculated the intensity distribution of the light field and the displacement parameters of the zoom lens system with different working distances. Figure 12 and Figure 13 show some of the intensity-distribution diagrams of light fields with working distances between 500–560 mm.

Secure .gov websites use HTTPS A lock ( Lock Locked padlock icon ) or https:// means you've safely connected to the .gov website. Share sensitive information only on official, secure websites.

Current beam-shaping methods mainly include aspherical-lens systems [4,5,6,7], diffractive optical elements [8], liquid-crystal spatial light modulators [9,10], and metasurfaces and metamaterials [11,12]. Aspherical cylindrical lenses are the most effective beam-shaping method for an intense laser beam-shaping system. This method has the advantages of a good shaping effect, low energy loss, and a simple structure. Additionally, only two aspherical cylindrical lenses are typically required to realize laser-beam expansion and shaping, and many previous studies have extensively investigated these applications. A Gaussian beam can effectively be shaped into a flat-top beam [13,14,15,16]; however, the shape of the beam spot cannot be changed.

Optics manufacturingcompanies

The optical structure diagrams of the focusing system in the Y- and X-directions are shown in Figure 6 and Figure 7, respectively. The geometrical dimensions of aspherical cylindrical lens 1, aspherical cylindrical lens 2, cylindrical lens 3, and cylindrical lens 4 are shown in Supplementary Materials.

There are some limitations in this paper; the laser-beam expansion and shaping system and focusing system are designed for a flat-top line-shaped beam spot with a length and width of 0.5 mm × 0.1 mm. For other sizes of flat-top line-shaped beam spots, it is necessary to design laser-beam expansion and shaping systems and focusing systems with different parameters. In the future, the beam expansion and shaping system and focusing system of the adjustable-size flat-top line beam spot will be studied.

Since only the flat-top Lorentz beam can obtain the analytical solution, the flat-top Lorentz function is used as the shaping objective. The shaping model of the flat-top Lorentz beam is shown in Figure 2.

We're dedicated to being the supplier you choose for your custom optic fabrication. From volume production to prototype, we will meet any require specifications at the most cost effective solution. Our other benefits include:

The magnification β = f2/f1, where f1 is the focal length of flat-concave lens and f2 is the focal length of flat-convex lens.

Optical lensmanufacturingprocess PDF

In Equation (1), r0 is the laser beam radius (mm) and I0 is the maximum light intensity (cd) of the laser beam. The light-field intensity distribution of the laser beam is shown in Figure 1.

The following supporting information can be downloaded at: https://www.mdpi.com/article/10.3390/s22114199/s1, Figure S1: Geometrical dimensions of aspherical cylindrical lens 1; Figure S2: Geometrical dimensions of aspherical cylindrical lens 2; Figure S3: Geometrical dimensions of cylindrical lens 3; Figure S4: Geometrical dimensions of cylindrical lens 4.

The data in Table 5 were linearly fitted to provide a motion-control mathematical equation for the dynamic focusing. The fitted data are shown in Table 6.

This study proposes a beam-shaping system based on aspherical cylindrical lenses. The proposed system uses the principle of the equivalent optical length of any beam between two aspherical lenses and the law of conservation of energy of the incident and outgoing laser beams to shape a circular laser spot with a Gaussian light-intensity distribution into a quasi-rectangular spot with a uniform intensity distribution. We introduce the design principle and method used for the shaping system through an example and analyze the effectiveness of the proposed method via a practical application.

When the working distance is between 500 and 535 mm, the intensity distribution of the light field exhibits a good rectangular flat-top beam. We set the working distance to a range of 500–535 mm; thus, we obtained a system scanning range of 2 L × 2 L, where

Along the cross-section of the Gaussian beam, the energy is concentrated around the spot center. To obtain a flat-top beam with uniform illumination, it is necessary to diverge the rays that pass through a small aperture and concentrate the rays that pass through a large aperture. Therefore, it is necessary to obtain the relationship between the coordinates of the rays on the entrance-pupil plane and those on the image plane, which is called the mapping function.

Optical Lensmanufacturingmachine

The simple physical model of the flat-top beam is represented by a circle function, which has the advantage of a simple form. However, it can only describe the uniform energy distribution of a flat-top beam and is unsuitable for calculating the beam transmission characteristics. Compared to other physical flat-top-beam models, the flat-top Lorentz model is the simplest for calculation. Therefore, the flat-top Lorentz model is selected as the physical model of the flat-top beam in this study to reduce the calculation complexity [17,18,19].

The depth of field of the focusing system is ±0.7 mm. Considering the systematic and random errors caused by the subsequent mechanical and electronic systems, we set the focusing error (working distance error) of the zoom lens system to ±0.1 mm, which is 1/7 of the total error.

Light-field-intensity distribution of the focusing system with a working distance of 500 mm. (a) Light-field-intensity distribution in the X-direction. (b) Light-field-intensity distribution in the Y-direction.

We offer FREE consultations and initial feasibility studies. If you have questions about limitations or lens capabilities we're more than happy to work through your proposed custom components. As an unmatched custom optical provider, we'll do everything that we can bring your vision to life.

The Galileo shaping system is composed of a flat concave lens and a flat convex lens, as shown in Figure 3. The convergence point generated by the Galileo-shaped structure is a virtual focus, which can avoid the air breakdown effect, and its axial size is smaller than that of the Kepler-shaped structure. Therefore, the application of the Kepler system for beam shaping requires laser power that is not too high, and the Galileo aspheric lens group can be applied to larger power.

Sample model 1 was used for multiple sintering experiments. When the Gaussian spot was used for the sintering experiment, the average deviation in the X-, Y-, and Z-directions were 0.35 mm, 0.50 mm, and −0.60 mm, respectively. When the flat-top line-shaped laser spot was used for the sintering experiment, the average deviation in the X-, Y-, and Z-directions were 0.30 mm, 0.40 mm, and −0.40 mm, respectively.

When the molded parts are placed horizontally, the overall molding efficiency is significantly improved. When the molded parts are placed sideways, the total scanning time of the galvanometer is similar to that achieved with horizontal placement. However, the powder bed fusion process requires more time, owing to the larger number of layers. Compared to the Gaussian laser, the scanning efficiency of the flat-top line-shaped laser is not considerably improved. When the number of layers is the same, a larger layer area results in a greater improvement in the scanning efficiency. The closer the placement angle of the molded part is to 45°, the lower the molding efficiency. However, as the layer area increases, the effect is smaller. It can be observed from the deviation rate of sample model 1 that the size deviations in the flat-top line-shaped beam and Gaussian beam are similar in the X- and Y-directions, and the size deviation in the flat-top line-shaped beam in the Z-direction is smaller than that of the Gaussian beam.

Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).

Part of our custom optical engineering solutions includes the utilization of the latest and most innovative technologies. Our team of experienced engineers use Zemax®, CODE V® and ASAP® for simulation and modeling techniques. Our network of mechanical engineers use SolidWorks® and Pro/ENGINEER® for custom optical design and complex COSMOS® to conduct thermal analysis and other simulations like diffraction light and stray light analysis.

Let the intensity of the incident light be Iin, the intensity of the outgoing light be Iout, the projection height of any ray on the incident plane be r1, and the corresponding projection height on the outgoing plane be r2. The beam–waist radius of the incident Gaussian beam is ω0, and the maximum radius is r0. The outgoing flat-top beam has a radius of R0. z1(r) and z2(r) are the shape functions of two aspheric surfaces. According to the law of conservation of energy, the energies contained in r1~r1 ± Δr1 and r2~r2 ± Δr2 are equal. The following normalization equations can be established in the Cartesian and polar coordinate systems:

No matter what kind of custom optical design you're looking for, our custom lens manufacturer will make your vision a reality. We provide custom objectives including deep ultraviolet applications to infrared photography, metrology, monitoring, microscopy and other custom optical lenses. Our projection lens designers and concept evaluation teams are the best at what they do.

Sample model 1 was placed at 0°, 30°, and 45° from the longest side in the X-axis direction, and the sintering experiment was performed five times. The scanning time of the galvanometer required to record the flat-top line-shaped spot and Gaussian spot are Ta1 and Ta2, respectively, and the times of the first and last scans are Tb1 and Tb2, respectively. We took the average of the results of the five experiments to obtain Ta1¯, Ta2¯, Tb1¯, and Tb2¯. The experimental results are shown in Table 8.

Considering the integrability of the outgoing flat-top beam, the flat-top Lorentzian function is used to express the intensity distribution as follows:

This study analyzed current laser-beam-shaping theory and systems and proposed a theory and system to produce non-imaging Gaussian laser beams and rectangular flat-top beam shaping. After discussing the laser beam-shaping theory of aspherical cylindrical lenses, we proposed the beam mapping function, called the flat-top Lorentzian function. Using ZEMAX, we designed the laser-beam expansion and shaping system and focusing system to mitigate the uneven beam energy when shaping a point light source into a surface light source. This study also analyzed the zoom lens system and it was observed that if the displacement of cylindrical lens 3 is precisely controlled, precise zooming can be achieved.

Three surfaces were inserted into the lens data editor (LDE). The second surface was set as a cylindrical surface, the glass material was set as ZnSe, and the thickness was set to 6 mm. The radius of the third surface was set to infinity. The radius of the second surface, conic, 4th, 6th, 8th, and 10th order coefficients, and the thickness of the third surface was set as optimization variables. The 2nd order system was omitted to reduce the processing complexity. The 4th, 6th, 8th, and 10th order coefficients were a4=−1.279×105, a6=2.878×107, a8=−2.878×109, and a10=1.25×1011, respectively.

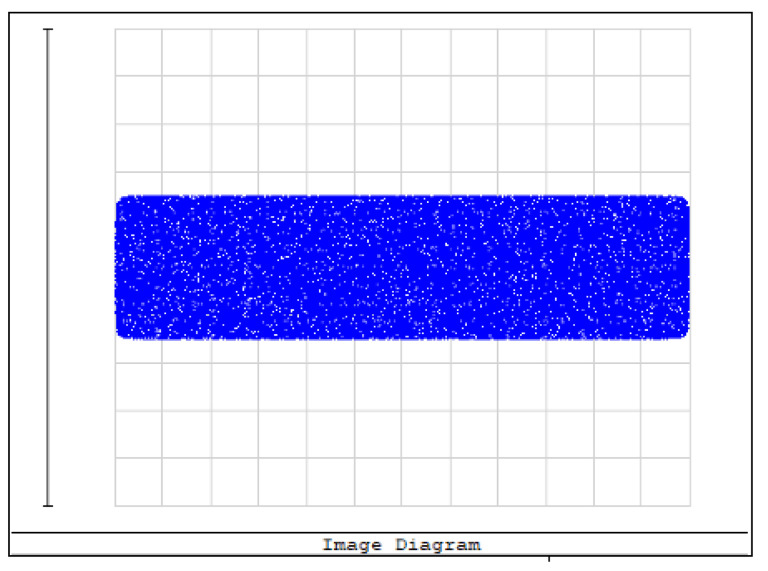
The light-field distribution of the flat-top rectangular beam combination optical system is shown in Figure 4. Figure 4a is the light-field intensity distribution in the X direction, and Figure 4b is the light-field intensity distribution in the Y direction. From the figure, the spot size in the X direction and Y direction is 60 mm and 15 mm, respectively, which meets the design requirements. Figure 5 shows the resulting light-field distribution spot diagram of the combined optical components on the X–Y plane, where each grid division represents 5 mm.

The method follows that of the previous sample model. Sample model 2 was placed at 0°, 30°, and 45° from the longest side along the X-axis. The sintering experiment was performed five times, and the scanning time of the galvanometer was recorded as Ta. The scanning times of the galvanometer required to record the flat-top line-shaped spot and Gaussian spot are Ta1 and Ta2, respectively. The times of the first and last scans are Tb1 and Tb2, respectively. We took the average of the results of the five experiments to obtain Ta1¯, Ta2¯, Tb1¯, and Tb2¯. The experimental results are shown in Table 9.

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations.

In this study, the circular Gaussian spot emitted by a laser light source is shaped into a rectangular flat-top beam to improve the scanning efficiency of a selective laser sintering scanning system. A CO2 laser with a power of 200 W, wavelength of 10.6 μm, and spot diameter of 9 mm is shaped into a flat-top spot with a length and width of 0.5 × 0.1 mm, and the mapping function and flat-top Lorentzian function are calculated. We utilize ZEMAX to optimize the aspherical cylindrical lens of the shaping system and the cylindrical lens of the focusing system. We then calculate the energy uniformity of the flat-top line-shaped beam at distances from 500 to 535 mm and study the zoom displacement of the focusing lens system. The results indicated that the energy uniformity of the flat-top beam was greater than 80% at the distances considered, and the focusing system must precisely control the displacement of the cylindrical lens in the Y-direction to achieve precise zooming.

The experimental results show that when the flat-top line-shaped laser spot is used for scanning and the placement angle is 0°, the scanning efficiency is approximately five-times that of the Gaussian spot. When the placement angle is 45°, the improvement in the scanning efficiency is at its lowest.

The laser scanning path adopted the alternate scanning modes of the X- and Y-axes, and the experimental molded parts were placed at 0°, 30°, and 45° from the longest side in the X-axis direction, as shown in Figure 17.

We're dedicated to being the supplier you choose for your custom optic fabrication. From volume production to prototype, we will meet any require specifications at the most cost effective solution. Our other benefits include:

The aperture, field of view, and wavelength were set similarly to the Y-direction, and a macro program was used to generate the evaluation function. In the macro program, the radius of the flat-top beam was changed (from 7.5 to 30 mm), and the operand was changed accordingly (from REAY to REAX). The rays converged in the X-direction; therefore, a coordinate-break surface was added to the LDE to rotate the cylindrical lens by 90° around the Z-axis. The 4th, 6th, 8th, and 10th order coefficients were a4=−4.816×105, a6=9.016×107, a8=−8.964×109, and a10=3.913×1011, respectively.

When the 4th order coefficient was used, the fitted nonlinear coefficient was a4=5.11633×106. The second aspherical lens was processed in the same way, and its aspheric coefficient was a4=5.25724×106.

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Conceptualization, C.L. and Y.G.; methodology, C.L.; software, C.L.; validation, C.L. and Y.G.; formal analysis, C.L.; investigation, C.L.; resources, Y.G.; writing—original draft preparation, C.L.; writing—review and editing, C.L. and Y.G.; visualization, C.L.; supervision, Y.G.; project administration, Y.G.; funding acquisition, Y.G. All authors have read and agreed to the published version of the manuscript.

The authors declare no conflict of interest. The founding sponsors had no role in the design of the study; in the collection, analyses, or interpretation of data; in the writing of the manuscript, and in the decision to publish the results.

The laser shaping and focusing system was tested using a selective laser sintering rapid prototyping machine (ASF 360, Longyuan AFS Co., Ltd., Beijing, China). Polystyrene powder produced by Longyuan AFS Co., Ltd. was used as the printing material. The flat-top line-shaped laser beam shaping system has an energy loss of approximately 17%; therefore, this study selected a 15 W Gaussian laser spot with a diameter of 0.1 mm and a 93 W flat-top line-shaped laser spot with a length and width of 0.5 mm and 0.1 mm, respectively, for experiments. The experimental parameters are shown in Table 7.

Opticalmanufacturingjobs

Alpha Industrial Park, Tu Thon Village, Ly Thuong Kiet Commune, Yen My District, Hung Yen Province Vietnam 17721 +84 221-730-8668 rfqvn@shanghai-optics.com

Taking the derivatives of Equations (12) and (13) and including an error of 0.1 mm in the equations, we obtain the following:

After substituting the function expressions of the Gaussian and flat-top Lorentzian beams into Equation (3), the mapping function can be obtained as follows:

Light-field intensity distribution of the combined optical system. (a) The light-field intensity distribution in X. (b) The light-field intensity distribution in Y.

EdmundOptics

We tested whether there was any deviation in the size of the experimental samples. We used a vernier caliper to measure and record the size of 27 experimental samples and calculated their size deviation in three directions.

We're proud to be a custom lens manufacturer that specializes in designing automated lighting systems, street lights and other indoors and outdoor lighting design systems. We also provide custom components, individual design and customized services to meet and exceed your expectations.

This research was funded by the Natural Science Foundation of Heilongjiang Province, grant number ZD2017009. This research was funded by the National Key Research and Development Program of China, grant number 2017YFD0601004.

Articles from Sensors (Basel, Switzerland) are provided here courtesy of Multidisciplinary Digital Publishing Institute (MDPI)

Shanghai Optics is an unparalleled custom optical engineering solution provider offering custom optical manufacturing, design and engineering services. We're one of the top custom lens manufacturers that specializes in custom lens assembly and delivers the best cost-effective custom optical solutions in the photonics industry. By combining high-quality custom components with the latest state of the art advanced metrology, our experts deliver breakthrough custom optical design solutions and engineering consulting for the toughest optical problems.

Optics manufacturingnear me

The Y-direction is consistent with the default coordinate setting in ZEMAX, and all coordinate systems in this study are the same as the default setting in ZEMAX. First, we set the wavelength and aperture. The aperture was set to 13.5 mm, and the field of view was set to 0.

Based on the above analysis, the displacement distance of cylindrical lens 3 is 0.5 mm, and the displacement error is less than ±0.0014 mm. The displacement distance of cylindrical lens 4 is 12.91 mm, and the displacement error is less than ±1.64 mm. Therefore, the zoom lens system can achieve precise zooming if the displacement distance of cylindrical lens 3 is well controlled.

In a selective laser sintering 3D-printing system, the emitted laser beam has a Gaussian energy distribution and circular spot. It also has a point-shaped Gaussian energy distribution after focusing; therefore, direct applications typically result in uneven heating and low-sintering-molding efficiency. To mitigate this limitation in practical applications, a laser beam with a circular spot and Gaussian energy distribution must be shaped into a rectangular spot with a flat-top energy distribution, and it should have a linear flat-top energy distribution after focusing [1,2,3]. This type of line-shaped laser irradiation sintering (line-shaped sintering) is equivalent to multiple lasers working simultaneously, and the resultant heating is uniform. This method can improve sintering quality and shorten the sintering time of molded parts. Moreover, in the process of laser shaping, the laser divergence angle is compressed to reduce the diffraction of the laser beam and obtain a thinner focusing line-shaped spot.

Light-field-intensity distribution of the focusing system with a working distance of 535 mm. (a) Light-field-intensity distribution in the X-direction. (b) Light-field-intensity distribution in the Y-direction.

Precisionoptics manufacturing

It is necessary to change the focal length of the focusing lens system during the scanning and molding process of the selective laser sintering system to realize the scanning of the processing surface. In the focusing lens system, aspherical cylindrical lenses 1 and 2 shape the beams in the X- and Y-directions, respectively. Two standard cylindrical lenses, cylindrical lens 3 and cylindrical lens 4, focus the beams in the X- and Y-directions, respectively. The optical-structure diagram is shown in Figure 11. The zoom function of the system can be achieved by changing the optical interval between aspherical cylindrical lens 2 and cylindrical lens 3 and that between aspherical cylindrical lens 2 and cylindrical lens 4. Therefore, cylindrical lenses 3 and 4 are defined as a zoom lens system.

The objective is to use aspherical cylindrical lenses to focus a rectangular flat-top beam with a size of 10 × 50 mm into a line-shaped light source with a size of 0.1 × 0.5 mm. The focus distance is 500 mm, and the glass material is ZnSe. Two aspherical shaping cylindrical lenses were designed as per the method described in Section 3.1. Based on the optimized data, the evaluation function was generated using a macro program, and the system was optimized. Table 2 shows the parameters of the optimized aspherical shaping cylindrical-lens-combined optical system. Table 3 shows the asphericity coefficient structural parameters of the optimized spherical cylindrical-lens-combined optical focusing system.

We performed a laser uniformity test on the designed optical system and used a CMOS beam analyzer (CinCam, CINOGY Technologies, Duderstadt, Germany) for testing. The diameter of the collimated Gaussian laser spot was approximately 9.01 mm, the size of the shaped rectangular flat-top spot was approximately 0.1 × 0.5 mm, and the laser energy in the spot was uniformly distributed.

Optics manufacturingprocess pdf

Official websites use .gov A .gov website belongs to an official government organization in the United States.

Room 609, 6/F, Global Gateway Tower, No.63 Wing Hong Street, Cheung Sha Wan, Kowloon, Hong Kong +852-54993705 info@shanghai-optics.com

By comparing Table 8 with Table 9, it can be concluded that when the flat-top line-shaped laser spot is used for scanning and the placement angle is 0°, the scanning efficiency is at its highest. When the placement angle is 45°, the scanning efficiency is at its lowest. Based on the data of sample models 2a and 2b, when the height of the Z-axis is 5 mm, the layer thickness is 0.1 mm, and the number of layers is 50, the non-working time of the galvanometer is similar. The larger the layer scanning area, the higher the scanning efficiency, and the smaller the influence of the placement angle.

Shanghai Optics design, manufacture and assemble custom lens systems/optical components from our global manufacturing facility. Learn about our capabilities, which includes diamond turning aspheric lens manufacturing, high laser damage threshold optical coating and state of the art metrology.

The placement is also an important factor that affects molding efficiency. Different placement methods affect the height of the molded part along the Z-axis. The larger the number of layers, the longer the non-working time of the galvanometer, and the lower the molding efficiency.

In Figure 1, ω0 is the waist radius of the Gaussian laser beam, defined as the radius of the laser beam when the peak light intensity drops to I0/e2.

An aperture diaphragm was placed 10 mm behind cylindrical lens 4. The size of the diaphragm was 20 × 4 mm, and its light-transmission efficiency was 84.472%. The flat-top distribution of the light field on the X–Y plane was more uniform after installing the diaphragm. The light-field-intensity distributions on the focal plane of the focusing system in the Y- and X-directions are shown in Figure 8 and Figure 9, respectively. The spot diagram of the light-field distribution on the X–Y plane is shown in Figure 10, where each grid division represents 0.05 mm.

In the selective laser sintering system, the molded parts are typically placed in one of three ways: horizontally, vertically, and sideways, as shown in Figure 14. Owing to the large volume of the parts, multiple layers and a long workbench scanning time are required to print the molded parts in the vertical direction. Therefore, this study only investigated the influence of the sideways placement and horizontal placement methods on the molding speed of the molded parts. Diagrams of the experimental samples are shown in Figure 15 and Figure 16, and each independent part is a 20 × 10 × 100 mm cuboid.

The optical beam expansion and shaping system based on aspherical cylindrical lenses can simultaneously adjust the intensity distribution and spot shape of the laser beam. The parameters of the incident light of the shaping object used in the system design are as follows: a CO2 laser is used with a power of 200 W, wavelength of 10.6 μm, and spot diameter of 9 mm. The Gaussian beam is shaped into a rectangular flat-top beam with a size of 15 × 60 mm using the aspherical cylindrical lenses. The working distance is 500 mm, and the glass material is ZnSe.

The nonlinear coefficient of the aspheric surface given in ZEMAX had a particular error; therefore, it was necessary to use the surface sag given in ZEMAX to refit the nonlinear coefficient of the surface. The sag data were fitted using Mathematica (MathWorks).

Equation (9) shows that when the flat-top Lorentzian function is used as a flat-top beam distribution function, its mapping function has an analytical solution, which can facilitate ray tracing and significantly simplify the numerical calculation process. For a Galilean-type aspheric system [20], there exists