which shows that the greatest magnification occurs for the lens with the shortest focal length. In addition, when the image is at the near-point distance and the lens is held close to the eye (\(ℓ=0\)), then \(L=d_i=25\,cm\) and Equation \ref{eq12} becomes

The condenser, composed of multiple lenses, is positioned below the stage of the microscope. Its role is to collect light from the light source, adjust the intensity of illumination, and ensure uniform brightness on the specimen. The aperture diaphragm, an important component within the condenser, allows control over the amount of light entering by adjusting its size, thus affecting the intensity of illumination and numerical aperture. A larger numerical aperture allows more light to enter, enhancing the quality of image formation. Different types of condensers can be chosen based on the type of specimen being observed. Bright-field condensers are used for observing transparent specimens, while dark-field condensers are used for observing opaque or semi-transparent specimens.  Specialized types such as phase contrast condensers and polarizing condensers are also available for specific observations.

This page titled 2.8: The Simple Magnifier is shared under a CC BY 4.0 license and was authored, remixed, and/or curated by OpenStax via source content that was edited to the style and standards of the LibreTexts platform.

Additionally, feel free to consult our professional sales team for assistance in selecting the most suitable microscope for you.

The apparent size of an object perceived by the eye depends on the angle the object subtends from the eye. As shown in Figure \(\PageIndex{1}\), the object at \(A\) subtends a larger angle from the eye than when it is position at point \(B\). Thus, the object at \(A\) forms a larger image on the retina (see \(OA′\)) than when it is positioned at \(B\) (see \(OB′\)). Thus, objects that subtend large angles from the eye appear larger because they form larger images on the retina.

A nosepiece is used for securing and switching between different magnification of objective lenses. Commonly, it comes in the type of triple nosepiece, quadruple nosepiece or quintuple nosepiece.

How to usemicroscope

where \(m\) is the linear magnification (Equation \ref{mag}) previously derived for spherical mirrors and thin lenses. Another useful situation is when the image is at infinity (\(L=\infty\)). Equation \ref{eq12} then takes the form

a. The required linear magnification is the ratio of the desired image diameter to the diamond’s actual diameter (Equation \ref{eq15}). Because the jeweler holds the magnifying lens close to his eye and the image forms at his near point, the linear magnification is the same as the angular magnification, so

What iseyepieceinmicroscope

The eyepiece is another crucial optical component installed within the microscope’s head or eyepiece tube. Its role is to further magnify the image produced by the objective lens. Common magnification levels for eyepieces include 10x, but depending on the observation needs, different magnifications such as 5x, 16x, 20x, etc., can be chosen. The field number of the eyepiece determines the field of view during observation, with a larger number indicating a wider field of view.

The illumination of a microscope provides uniform and adjustable lighting to the objective lens, resulting in clearer imaging. The main types of light sources include halogen, mercury, and LED lamps. Halogen lamps produce light close to natural light and are relatively inexpensive, suitable for most microscopes, but they generate a significant amount of heat and have a relatively short lifespan. Mercury lamps offer high brightness and can provide a powerful source of ultraviolet light, making them suitable for fluorescence microscopes, but they also have a short lifespan. LEDs have high efficiency, low heat generation, and can produce bright and uniform light sources, but they tend to be relatively more expensive. Different observations and adjustments are determined by another optical component called the condenser.

From Figure \(\PageIndex{1b}\), we see that the absolute value of the image distance is \(|d_i|=L−ℓ\). Note that \(d_i<0\) because the image is virtual, so we can dispense with the absolute value by explicitly inserting the minus sign:

Note that a greater magnification is achieved by using a lens with a smaller focal length. We thus need to use a lens with radii of curvature that are less than a few centimeters and hold it very close to our eye. This is not very convenient. A compound microscope, explored in the following section, can overcome this drawback.

Note that all the quantities in this equation have to be expressed in centimeters. Often, we want the image to be at the near-point distance (e.g., \(L=25\,cm\)) to get maximum magnification, and we hold the magnifying lens close to the eye (\(ℓ=0\)). In this case, Equation \ref{eq12} gives

Depending on their application, optical microscopes can be categorized into various types such as biological microscopes, stereo microscopes, fluorescence microscopes, and metallurgical microscopes.

\[\begin{align} M&= \left(−\dfrac{d_i}{d_o}\right)\left(\dfrac{25\,cm}{L}\right) \\[4pt] &=−d_i\left(\dfrac{1}{f}−\dfrac{1}{d_i}\right)\left(\dfrac{25\,cm}{L}\right) \\[4pt] &= \left(1−\dfrac{d_i}{f}\right)\left(\dfrac{25\,cm}{L}\right) \label{eq10} \end{align} \]

b. To get an image magnified by a factor of ten, we again solve Equation \ref{eq13} for \(f\), but this time we use \(M=10\). The result is

Electron microscopes use electron beams instead of visible light and come in two types: transmission electron microscopes (TEM) and scanning electron microscopes (SEM). They have similar basic components, including an electron source, lens system, vacuum system, and sample chamber. Building upon the foundation of optical microscopes, which have a resolution of 0.2μm, electron microscopes boast an impressive resolution of 0.2nm. This means they can magnify objects much more than optical microscopes, with an overall magnification reaching up to 200,000X. They are widely used for studying the shapes of molecules and atoms and in the field of nanotechnology. However, because samples must be observed in a vacuum, electron microscopes cannot be used to observe live samples, and they also come with higher purchasing and maintenance costs.

We use cookies to enhance your browsing experience, serve personalized ads or content, and analyze our traffic. By clicking "Accept", you consent to our use of cookies.

\[\underbrace{ M=\dfrac{θ_{image}}{θ_{object}}=\dfrac{h_i(25cm)}{Lh_o}}_{\text{angular magnification}} . \label{angular magnification} \]

Eyepiecelensmicroscope

Consider the situation shown in Figure \(\PageIndex{1b}\). The magnifying lens is held a distance \(ℓ\) from the eye, and the image produced by the magnifier forms a distance \(L\) from the eye. We want to calculate the angular magnification for any arbitrary \(L\) and \(ℓ\). In the small-angle approximation, the angular size \(θ_{image}\) of the image is \(h_i/L\). The angular size \(θ_{object}\) of the object at the near point is \(θ_{object}=h_o/25\,cm\). The angular magnification is then

Focusing consists of coarse and fine adjustments. It is typically located on the side of the arm. First, select the low-power objective lens and use the coarse adjustment knob to bring the specimen into view. Then, switch to the high-power objective lens and use the fine adjustment knob to obtain a clear image.

Functionof objective lens inmicroscope

The stage is used to hold the specimen being observed, securing it with clips, and allowing movement of the specimen in the X-Y axes for precise positioning. Some advanced microscopes are equipped with motorized stages, which can enhance efficiency and observation accuracy.

Inserting Equation \ref{eq34} into Equation \ref{eq10} gives us the final equation for the angular magnification of a magnifying lens:

Functionof body tube inmicroscope

To account for the magnification of a magnifying lens, we compare the angle subtended by the image (created by the lens) with the angle subtended by the object (viewed with no lens), as shown in Figure \(\PageIndex{1a}\). We assume that the object is situated at the near point of the eye, because this is the object distance at which the unaided eye can form the largest image on the retina. We will compare the magnified images created by a lens with this maximum image size for the unaided eye. The magnification of an image when observed by the eye is the angular magnification \(M\), which is defined by the ratio of the angle \(θ_{image}\) subtended by the image to the angle \(θ_{object}\) subtended by the object:

The microscope was invented by the Dutch eyeglass merchant Zacharias Janssen in the late 16th century. This invention broke the limitations of human observation confined to the naked eye. Through one or more lenses, the microscope can magnify and observe tiny objects that are invisible to the naked eye. As a precision instrument, the microscope has been widely applied in fields such as education, medicine, biology, geology, materials science, forensic science, and electronic inspection.

Functionof diopter adjustment inmicroscope

Microscopes come in many types, but the term “microscope” commonly refers to an optical microscope in everyday language. Optical microscopes use lenses to magnify objects. The magnification is determined by multiplying the magnification of the objective lens by that of the eyepiece. Currently, most microscopes can achieve magnifications up to 1000X. Due to limitations in resolution, 1600X is considered the maximum magnification for optical microscopes. Resolution is limited by the wavelength of the optical system, which means that beyond a certain magnification, lenses cannot clearly distinguish smaller details.

After understanding the basic concepts of microscopes, there are several factors to consider when choosing the most suitable microscope from various types available in the market:

Magnification and resolution are crucial factors of microscopes. Choose the appropriate magnification based on the type of specimen you need to observe.

In our article: How Many Types of Optical Microscopes? We will provide detailed descriptions of the working principles and applications of each type. Optical microscopes are relatively easy to operate and have certain advantages in terms of price. That makes them a good choice for beginners.

We need to determine the requisite magnification of the magnifier. Because the jeweler holds the magnifying lens close to his eye, we can use Equation \ref{eq13} to find the focal length of the magnifying lens.

What is thefunctionof arm inmicroscope

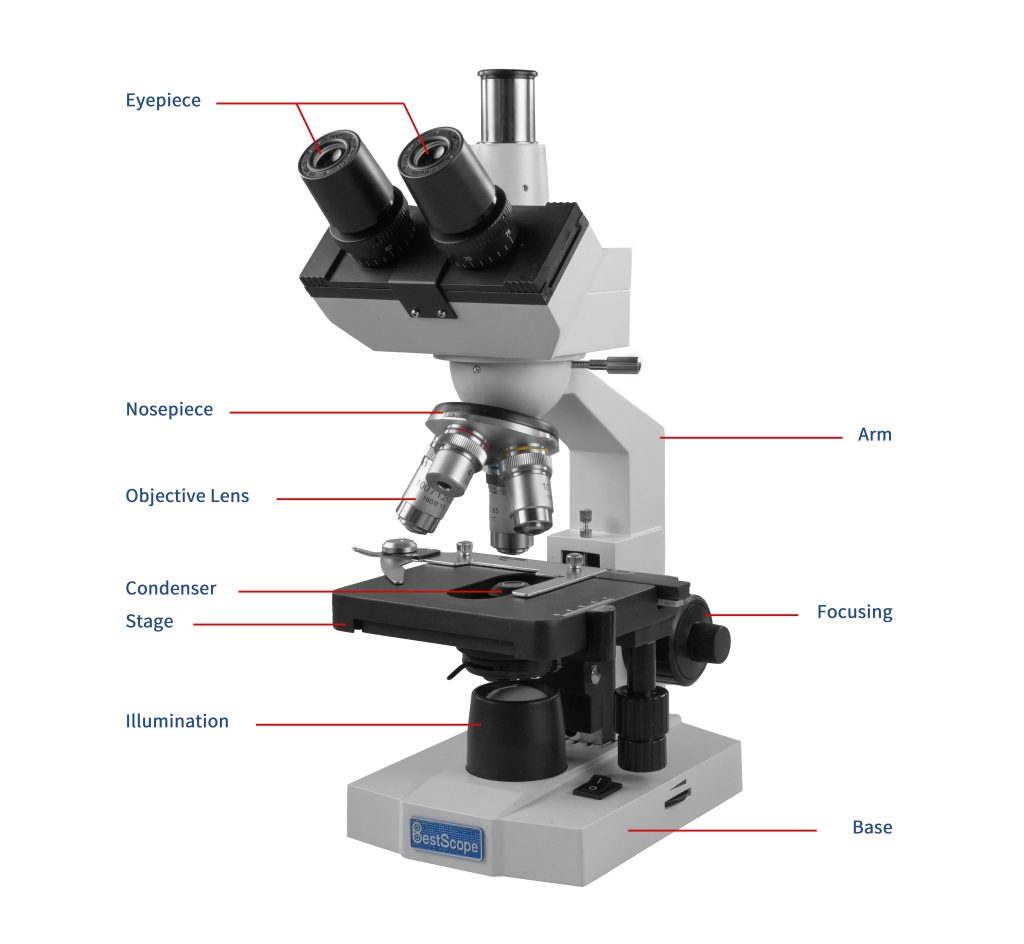
The objective lens is a crucial component of a microscope. It is made up of multiple lenses. Its main function is to magnify the specimen at different levels. Common magnification levels include 4x, 10x, 40x, and 100x. The 100x high-power objective lens typically requires immersion oil for use. It has a lower working distance, and requires more careful handling to avoid damaging the specimen or the lens. In addition to magnification and working distance, numerical aperture (NA) is also an important parameter of the objective lens, determining its optical performance and resolution. The larger numerical aperture brings the higher resolution, which allows for clearer observation of specimens.

Cost is also a necessary factor to consider when purchasing a microscope. Find a cost-effective microscope that combines your budget. Remember, the highest-priced microscope may not always be the best choice. Instead, focus on selecting a microscope with good optical performance and durability that meets your requirements.

A jeweler wishes to inspect a 3.0-mm-diameter diamond with a magnifier. The diamond is held at the jeweler’s near point (25 cm), and the jeweler holds the magnifying lens close to his eye.

Microscopeparts and functions

For inspecting circuit boards, small components, or studying insects where high magnification is not required, a stereo microscope is preferred.

The resulting magnification is simply the ratio of the near-point distance to the focal length of the magnifying lens, so a lens with a shorter focal length gives a stronger magnification. Although this magnification is smaller by 1 than the magnification obtained with the image at the near point, it provides for the most comfortable viewing conditions, because the eye is relaxed when viewing a distant object.

By comparing Equations \ref{eq13} and \ref{eq15}, we see that the range of angular magnification of a given converging lens is

The LibreTexts libraries are Powered by NICE CXone Expert and are supported by the Department of Education Open Textbook Pilot Project, the UC Davis Office of the Provost, the UC Davis Library, the California State University Affordable Learning Solutions Program, and Merlot. We also acknowledge previous National Science Foundation support under grant numbers 1246120, 1525057, and 1413739. Legal. Accessibility Statement For more information contact us at info@libretexts.org.

We have seen that, when an object is placed within a focal length of a convex lens, its image is virtual, upright, and larger than the object (see part (b) of this Figure). Thus, when such an image produced by a convex lens serves as the object for the eye, as shown in Figure \(\PageIndex{2}\), the image on the retina is enlarged, because the image produced by the lens subtends a larger angle in the eye than does the object. A convex lens used for this purpose is called a magnifying glass or a simple magnifier.