The other characteristics of an optical system, such as a lens, are the f-number and the focal length. The focal length is the distance over which the initially collimated light rays are brought to focus. The larger the focal length, the more distant objects can be seen sharply. You can check the thin lens equation or lens-maker equation calculators to study how to compute the focal length of a lens.

F-stops

This also demon­strates that an increase in focal length decreas­es the inten­si­ty of light reach­ing the image sen­sor. How­ev­er, because aper­tures are cal­i­brat­ed pro­por­tion­ate­ly to focal length, all lens­es set to the same f‑stop will the­o­ret­i­cal­ly trans­mit the same amount of light regard­less of focal length. The equa­tion con­sid­ers every­thing and pro­vides an aper­ture f‑stop that rep­re­sents equal light inten­si­ty across all lens­es.

Hyperfocal distance

Physicist at the Institute of Nuclear Physics in Kraków interested in magnetism and always ready to explain everything to everyone in simple terms. He is currently working on adding more scientific papers to his collection, accompanied by his son and another baby on its way. A perfectionist with an acute eye for detail, he has a unit converter in his brain and uses it to compare prices at the supermarket. Loves peace and quiet, especially during hiking. See full profile

This aperture area calculator helps you to compute the aperture area of a lens. Try the calculator right now, or keep reading to learn about the aperture diameter, f-number, and the aperture area equation.

Focal length

Since no lens spec­i­fies the diam­e­ter of its entrance pupil, but every lens pro­vides you with focal length and f‑stop val­ues, we can express the equa­tion as D=ƒ/N. For exam­ple, take a 50 mm lens set to ƒ/4, and you obtain an entrance pupil of 12.5 mm. Set the same lens to ƒ/1.4, and the entrance pupil becomes 35.7 mm. Now, the rea­son for the unin­tu­itive inverse rela­tion­ship of f‑stop num­bers and aper­ture size is appar­ent: the entrance pupil can only be larg­er when set­ting the aper­ture to a small­er f‑stop.

Fstops explained

The eye is the most fit­ting ana­logue for under­stand­ing the struc­ture and func­tion of a lens aper­ture. The iris of an eye reg­u­lates the retina’s expo­sure to light by dilat­ing or con­tract­ing the pupil. Inside a cam­era lens, a large aper­ture is sim­i­lar to an enlarged pupil; it rais­es expo­sure by increas­ing the flow of light. A small aper­ture is like a con­tract­ed pupil; it reduces expo­sure by decreas­ing light flow.

Aper­ture size set­tings are indi­cat­ed by f‑numbers, also known as f‑ratios or f‑stops (the lat­ter used most com­mon­ly in pho­tog­ra­phy lin­go). They are expressed with the hooked f fol­lowed by a num­ber, such as ƒ/2, ƒ/5.6, and so on. Each f‑stop cor­re­sponds to a spe­cif­ic aper­ture size in each lens. The range of pos­si­ble f‑stops varies depend­ing on the lens. The stan­dard series of f‑stops is:

For example, if we set the f-number to be 1.4 for a standard lens of a focal length f = 70 mm, then the aperture diameter is D = 50 mm. Using the aperture area calculator, we find that the aperture area is A = 1963.3 mm².

The f‑number is a ratio of the lens focal length divid­ed by the diam­e­ter of the entrance pupil, also known as the effec­tive aper­ture. (An entrance pupil is the image of the aper­ture seen through the lens’s front.) The equa­tion is N=ƒ/D, where N is the f‑number, ƒ the lens focal length, and D is the entrance pupil diam­e­ter. For exam­ple, a lens with a 50 mm focal length and an entrance pupil diam­e­ter of 25 mm would have an aper­ture of ƒ/2 (50÷25=2). A lens with a 100 mm focal length and an entrance pupil diam­e­ter of 50 mm would also have an aper­ture of ƒ/2 despite hav­ing an entrance pupil diam­e­ter that’s twice as large.

f-stop是什么

Aperture area is the area associated with the light-collecting section of an optical system. Larger values correspond to more light entering, brighter images, and greater focal lengths, whereas smaller ones translate into darker images and shorter focal lengths.

In commercially produced lenses, we can usually set the f-number to some prescribed values like 1.4, 2, 2.8, 4, 5.6, ... . They correspond to decreasing the aperture diameter by a factor of √2. In turn, the aperture area decreases by a factor of 2.

Numerical aperture

An aperture is a hole, or an opening, in an optical system through which the light enters. The larger the aperture, the more light can enter. At the same time, the light is less collimated. Smaller aperture results in more collimated light entering at the cost of lower intensity. If you want to learn about different ways of measuring light intensity, check the lumen calculator. The aperture diameter is just the diameter of the opening.

F‑numbers have an inverse rela­tion­ship to the aper­ture size: a low­er f‑number indi­cates a big­ger aper­ture that col­lects a con­sid­er­able vol­ume of light; a high­er f‑number means a small­er aper­ture that gath­ers less light. For instance, adjust­ing your aper­ture set­ting from ƒ/4 to ƒ/2.8 dou­bles your expo­sure (adds 1 EV); shift­ing from ƒ/4 to ƒ/5.6 halves your expo­sure (sub­tracts 1 EV); going from ƒ/5.6 to ƒ/2 increas­es expo­sure by three stops (adds 3 EV), which is eight times more light (2×2×2=8). Many begin­ners find this rela­tion­ship unin­tu­itive, even con­fus­ing; the fol­low­ing mnemon­ic device could help you mem­o­rize the inverse con­nec­tion (adapt­ed from Dig­i­tal Pho­tog­ra­phy for Dum­mies):

f-stop app

Adena Benn is an Information Technology teacher with a degree in Computer Science. She has been teaching high schoolers since 1996 and loves everything computers. She loves problem-solving, so creating calculators to solve real-world problems is her idea of fun. She writes children's stories, is a seamstress, and is an avid reader. In her spare time, she travels and enjoys nature. See full profile

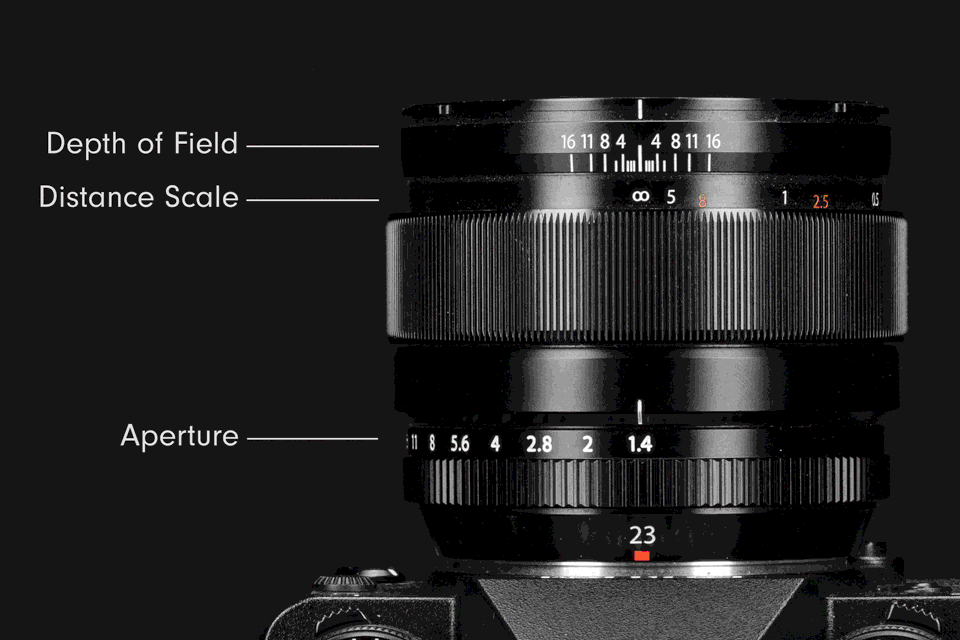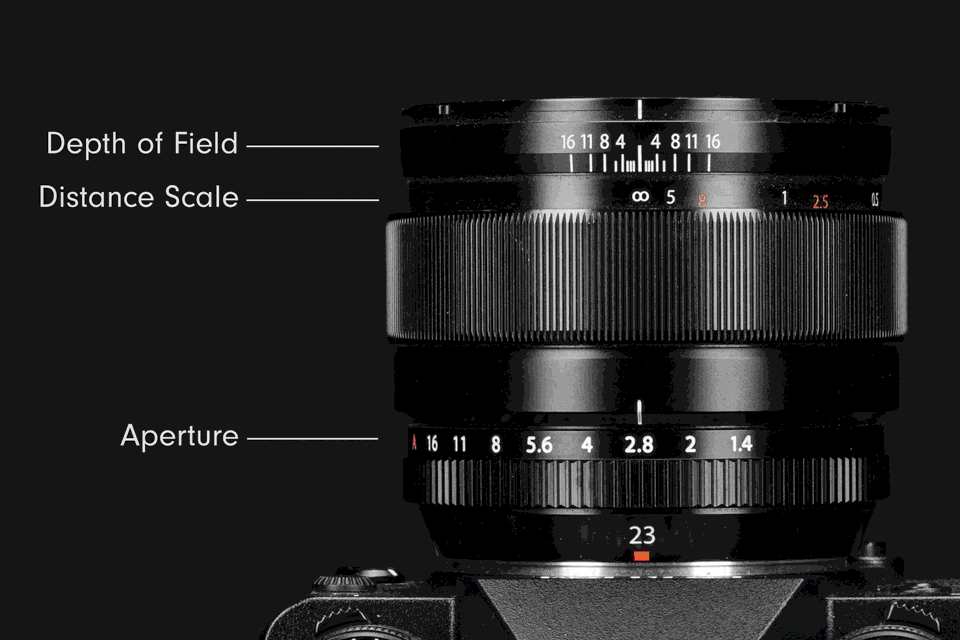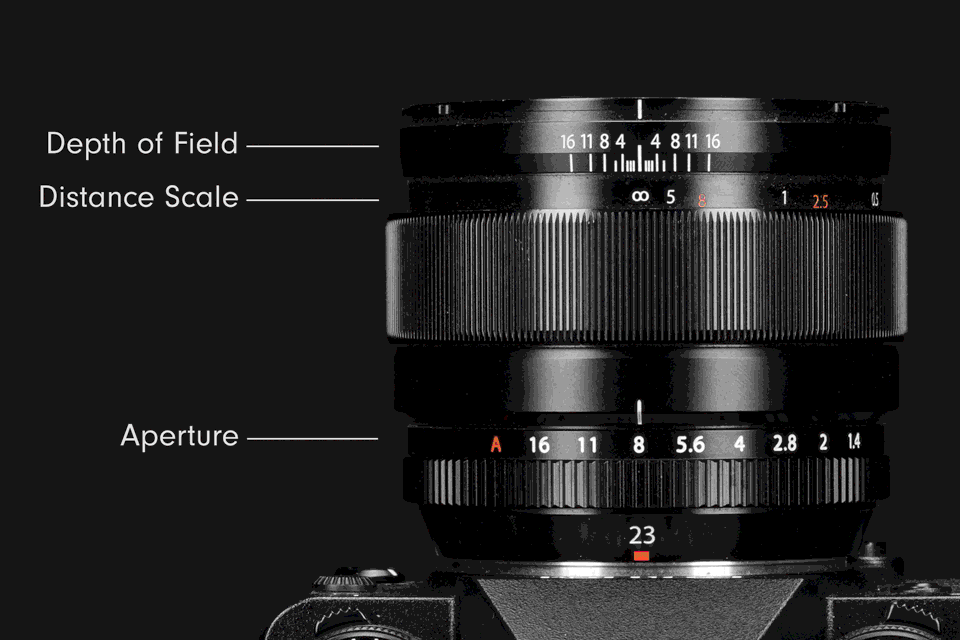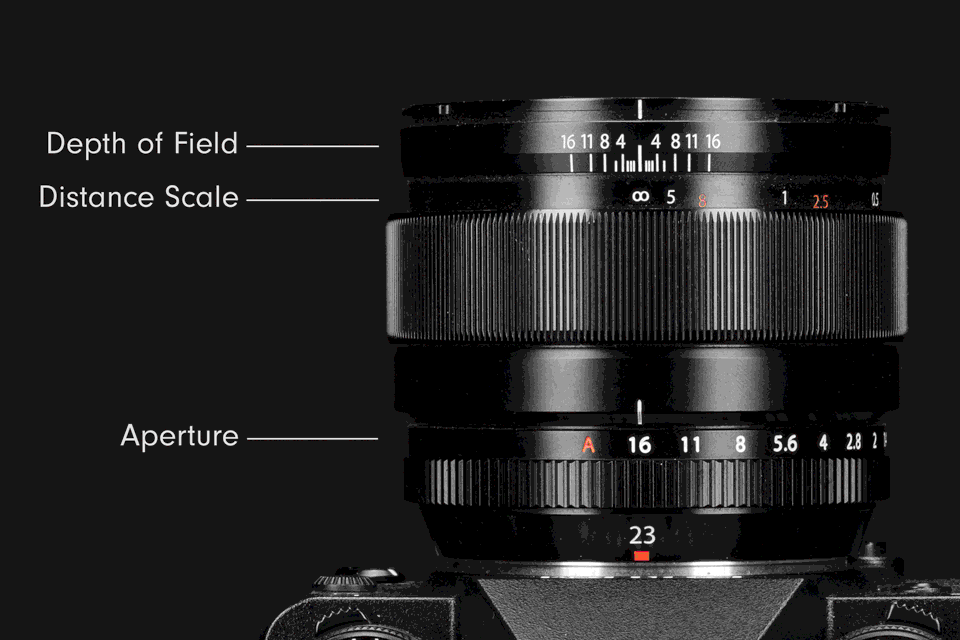
They are con­sid­ered stan­dard f‑stops for two rea­sons: the dif­fer­ence in expo­sure when shift­ing between adja­cent num­bers is equal to one stop (1 EV); tra­di­tion­al­ly, lens­es with phys­i­cal aper­ture selec­tion rings would indi­cate the avail­able aper­ture range using these stepped inter­vals. Most cam­eras per­mit the selec­tion of inter­me­di­ate f‑stops in one-third-stop incre­ments.

Inside a lens is a ring of over­lap­ping blades col­lec­tive­ly known as an iris diaphragm, or iris. The expan­sion or con­trac­tion of the iris blades adjusts the size of the open­ing at its cen­tre, which is called an aper­ture. Chang­ing the size of the aper­ture con­trols the inten­si­ty of light pass­ing through the lens.

The microscope's aperture area is 113.1 mm2. You can easily find this value by substituting the diameter (D = 12 mm) into the aperture area equation: